Gabriel Barceló Summary: The object of our study is the behavior of rotational rigid bodies. Although we barely realize, we liv...
Gabriel Barceló
Summary: The object of our study is the behavior of rotational rigid bodies.
Although we barely realize, we live in a physic world in which everything rotates, a world with intrinsic movement in symmetrical axes.
In this universe, which is characterized by a rotational dynamic behavior, the scientific thought has mainly developed a dynamic based on the lineal translation. This is, therefore, contradictory. In our opinion, this dynamic is not always applicable to bodies with angular momentum.
Traditionally, we have believed that the Earth was a flat surface where we were subjected exclusively a dynamics based on lineal translations. In fact, we find ourselves in a stage formed by bodies in constant rotation and orbiting: the Earth is rotating while it orbits; however, the Sun rotates while it is moving drawing its own trajectory around our galaxy.
GJRE: Global Journals Blog
From determinate dynamics assumptions and based on a new interpretation of the bodies with intrinsic angular momentum, when they are supporting successive non-coaxial torques, we have developed new dynamics hypothesis which allow us to reach the conclusion to create a new mathematical model in the rotation dynamics fields.
This new model would allow us to justify dynamic natural behaviors that until now have not been sufficiently understood. With this new conceptual model, new results for determinate cases are obtained based on a new interpretation of the composition of movements originated by external momentums.
We think that the obtained results allow us to have a new dynamic point of view which was unknown until now. This new dynamic perception gives us the possibility of transforming the considered chaotic trajectories into other deterministic and modelling trajectories. We have concluded that a new scientific field exists. However it has not been structured in dynamic terms and, specifically, in rigid solid bodies with multiple non coaxial simultaneous rotations.
For this goal, we have deducted that analysis is necessary of the velocity and acceleration fields which are generated in bodies with intrinsic angular momentum. It is necessary as well to consider new coupling criteria of the dynamics magnitude. In this context, ddynamic reactions and inertial fields appear, and they cannot be justified by Classical Mechanics.
We have aimed to develop these researches and to inform about the surprising obtained results. We have also the objective to apprehend the interest of this new area of knowledge: the rotational dynamics and its multiple and remarkable scientific and technological applications.[1]
We have not found any scientific literature along the 20th and 21st centuries with similar studies on rigid solid bodies supporting external actions which generate spatially mismatched simultaneous accelerations; therefore we believe that our study is innovative and the proposed conclusions have not been presented up till now.
One conclusion of our analysis is the suggestion that in some cases the matter is unable to add vertorially the angular momentum of rotational bodies.
Classical Mechanics has been formulated for inertial references and it does not say anything and it does not relate to rotation cases. Nevertheless, creating a mechanics available for any stage is possible if we include their inertial reactions and we define a Fields Inertial Dynamic. In this the inertial phenomena would be structured rationally, and incorporate a unified mechanics.
In order to include the inertial phenomena in the physics knowledge structure, it is necessary to analyze the movement in non holonomous coordinates and the axial reactions which are produced. We understand that a classical mechanics based on holonomous coordinates and polar reactions, will only represent a limited and partial natural view.
The proposed generalization does not say that the classical mechanics is outdated or wrong, but it that it is limited and partial, because it is only referred to inertial systems. We can be more aspiring and search more general dynamical laws which regulate the movement bodies behavior under rotations or, supporting multiple and non-coaxial rotations.
The Theory of Dynamic Interactions generalizes the gyroscopic couple concept and other inertial phenomena concepts and it includes into a unified structure of the new rotational non inertial dynamic.
According to the Theory of Dynamic Interactions we can understand a new universe in constant dynamic balance where, the torque which results null, will generate, a constant orbiting in a close trajectory. This mathematical model is very relevant. In this model, the principal effects are the forces and also the torques. This effect will produce orbital motions, and repetitive movements, generating a balance dynamical system and not on limited expansion.
Although we barely realize, we live in a physic world in which everything rotates, a world with intrinsic movement in symmetrical axes.
In this universe, which is characterized by a rotational dynamic behavior, the scientific thought has mainly developed a dynamic based on the lineal translation. This is, therefore, contradictory. In our opinion, this dynamic is not always applicable to bodies with angular momentum.
Traditionally, we have believed that the Earth was a flat surface where we were subjected exclusively a dynamics based on lineal translations. In fact, we find ourselves in a stage formed by bodies in constant rotation and orbiting: the Earth is rotating while it orbits; however, the Sun rotates while it is moving drawing its own trajectory around our galaxy.
GJRE: Global Journals Blog
From determinate dynamics assumptions and based on a new interpretation of the bodies with intrinsic angular momentum, when they are supporting successive non-coaxial torques, we have developed new dynamics hypothesis which allow us to reach the conclusion to create a new mathematical model in the rotation dynamics fields.
This new model would allow us to justify dynamic natural behaviors that until now have not been sufficiently understood. With this new conceptual model, new results for determinate cases are obtained based on a new interpretation of the composition of movements originated by external momentums.
We think that the obtained results allow us to have a new dynamic point of view which was unknown until now. This new dynamic perception gives us the possibility of transforming the considered chaotic trajectories into other deterministic and modelling trajectories. We have concluded that a new scientific field exists. However it has not been structured in dynamic terms and, specifically, in rigid solid bodies with multiple non coaxial simultaneous rotations.
For this goal, we have deducted that analysis is necessary of the velocity and acceleration fields which are generated in bodies with intrinsic angular momentum. It is necessary as well to consider new coupling criteria of the dynamics magnitude. In this context, ddynamic reactions and inertial fields appear, and they cannot be justified by Classical Mechanics.
We have aimed to develop these researches and to inform about the surprising obtained results. We have also the objective to apprehend the interest of this new area of knowledge: the rotational dynamics and its multiple and remarkable scientific and technological applications.[1]
We have not found any scientific literature along the 20th and 21st centuries with similar studies on rigid solid bodies supporting external actions which generate spatially mismatched simultaneous accelerations; therefore we believe that our study is innovative and the proposed conclusions have not been presented up till now.
One conclusion of our analysis is the suggestion that in some cases the matter is unable to add vertorially the angular momentum of rotational bodies.
Classical Mechanics has been formulated for inertial references and it does not say anything and it does not relate to rotation cases. Nevertheless, creating a mechanics available for any stage is possible if we include their inertial reactions and we define a Fields Inertial Dynamic. In this the inertial phenomena would be structured rationally, and incorporate a unified mechanics.
In order to include the inertial phenomena in the physics knowledge structure, it is necessary to analyze the movement in non holonomous coordinates and the axial reactions which are produced. We understand that a classical mechanics based on holonomous coordinates and polar reactions, will only represent a limited and partial natural view.
The proposed generalization does not say that the classical mechanics is outdated or wrong, but it that it is limited and partial, because it is only referred to inertial systems. We can be more aspiring and search more general dynamical laws which regulate the movement bodies behavior under rotations or, supporting multiple and non-coaxial rotations.
The Theory of Dynamic Interactions generalizes the gyroscopic couple concept and other inertial phenomena concepts and it includes into a unified structure of the new rotational non inertial dynamic.
According to the Theory of Dynamic Interactions we can understand a new universe in constant dynamic balance where, the torque which results null, will generate, a constant orbiting in a close trajectory. This mathematical model is very relevant. In this model, the principal effects are the forces and also the torques. This effect will produce orbital motions, and repetitive movements, generating a balance dynamical system and not on limited expansion.
2. Source of inspiration
It is possible in nature to find simultaneous intrinsic rotation and orbiting movements. However, a
physical or mathematical model which explains a scientific correlation between both movements
when they appear simultaneously does not exist. It is strange. Also, the existence of a constant
coincidence between the orbit simultaneity and spin in atomic physics is relevant.
However, the existence of these physical and mathematical relations between both simultaneous movements of rotation and orbiting could be proposed intuitively.
Moreover, we can ask if the actual dynamic laws provided to define the behavior of the bodies in the space, have enough precision or they do not reflect exactly all possibilities in our world.
We do not know about other type of analysis which study both simultaneous phenomena and could determine a possible interaction between both phenomena. But the fact is that in universe, all orbiting body has intrinsic rotation.
For this reason, I decided to investigate if any physical correlation between both movements exists and, in that case, I would try to obtain its mathematical formulation.
If it were true, the dynamic laws which have been accepted in order to determine the rotational bodies’ behavior until now, would be insufficient to describe the physical reality of the rotational bodies’ movements exactly.
In our study, we research non inertial systems in order to better understand the behavior of rigid bodies supporting non coaxial and simultaneous rotations. As the result of this analysis, I have suggested new hypotheses which oblige to apply these studies to the theory of fields with the objective to explain the dynamic of those bodies.
With this explanation provided, the observation of the universe caused new questions: its secular dynamic balance does not seem to adapt to a Newtonian physics in which the force cause translational accelerated movements continually. The universe balance and its dynamic, does not seem to sympathize with this conceptual structure of classical mechanics.
The analysis of the superposition and composition of the simultaneous movements were done by Galileo [2] in order to explain the trajectory of a cannonball. The understanding or correct interpretation of the fields superposition with dynamic magnitudes generated by momentums or torques, is essential to analyze these phenomena correctly.
In my studies, I have researched the movement of the objects which are supporting multiple rotations, like the spinning top, the boomerang, the hoop and others whose peculiar behavior has been intriguing to the human mind. I think that the conclusions that I show in my texts, change the rational dynamic bases, and incorporate new significant and high impact criteria in rotational dynamics.
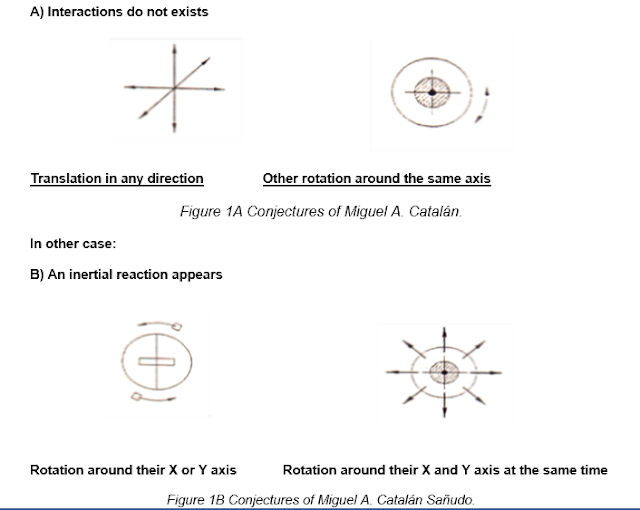
Around 1956, the scientist and professor Miguel Catalán [3] proposed to his students, some conjectures about the rotational dynamic by observing the gyroscope behavior and its reactions [4]. In his text books he proposed a possible correlation between the atom structure and the rotational classical dynamic. These conjectures generated in me a scientific curiosity during many years.[5]
Catalán suggested us to experiment with a gyroscope whose rotor had constant rotation around its Z axis. He invited us to take this device with only one hand and perform the following movements:
The results of these experimental proofs are the following:
1. Translation in any direction:
we take a gyroscope with our hand when it is rotating and we move it in a straight line in different directions, maintaining its axis parallel to Z axis or the principal gyroscope axis. No interaction reaction is produced (Look at Figure 0.1A).
2. Additional rotation around the rotation axis: if the device is also rotated around its rotation Z axis, no interaction appears. (Look at Figure 0.1A)
3. Rotation around other axis: if the gyroscope is rotated around any other axis, a resistance to movement is felt in the hand that supports it. (Look at Figure 0.1B)
After these observations, professor Catalán explained us that, in that moment physics had not the answer for these strange phenomena in which we recognized a force which acts on our hand, generating and evident resistance to displacement when we executed a simple rotational movement around any different axis to Z axis.
Our initial speculation and conjectures appeared for the first time in those years, when we endeavored to understand the true dynamic behavior in the rigid solid bodies in non inertial systems (See figure 0.2):
With all these questions in mind, I started in the early eighties of the last century, an exhausting historic study on rotational classical dynamics, to get to know the background and what had been written about the subject and other related issues like inertia, magnitude conservation, etc.
I was able to verify that some studies initiated in the nineteenth century, had been later on forgotten. For example, Esprit Jouffret (1831-1904), in the late nineteenth century,7 had already started to analyze the dynamic of the caused reactions in a particle of a rigid body, when it is supported successive non coaxial torque, and M. Hirn8 had concluded that in these situations, non- homogeneous distributions speeds are generated.
As a result of this historical study, I carried out a compendium of all these studies and an abstract, which I published like a summary book about the rotational classical dynamic.9 In this book the different published works which had referred to rotational bodies during the Physics history, were analyze analyzed and commented.
Later on, I defined some differentiated dynamic hypothesis which suggested a rotational alternative dynamic. From these new hypotheses, I established a new specific mathematical formulation in order to determine the movement equations of these bodies by doing experimental positive testing in order to confirm these initial hypotheses.
Therefore, the origin of the Theory of Dynamic Interactions (TDI), arises from the physical observation and the reflection on the validity of mathematical models which accept the vectorial addition of angular magnitudes, with no differentiation on their priority:
Some behavior laws [12] were deduced and suppositions and application phenomena were conceived in addition to the experimental testing which has been done. With a conceptual structure confirmed, I published a new text [13] in which I showed, for the first time, the developed theory and some scientific articles.[14]
3.How much time spent
Although our physics professor, Miguel Catalán managed to pass on to me this curiosity and the dream excitement of the study of physical phenomena, over sixty years ago; it has been for the last thirty-five years that, I have managed to carry out a systematic investigation with a group of collaborating friends. The purpose has been throughout the better understand of the behavior of rigid solid bodies simultaneously submitted to non-coaxial rotations, over the latest 35 years. This research has allowed us to propose new hypothesis about the field’s theory in order to explain the dynamic behavior of the bodies which support non-coaxial accelerations.
Our initials goals in this project were (look at figure 3):
In our experimental line and logical argumentation, in the first place we studied the concept of rotation in Classical Mechanics, but also in Einstein's studies and in the modern theories of relativity. Upon completion of this analysis, we concluded that proposing new specific hypothesis for the movements of the intrinsic rotational bodies is possible. For that purpose, we only needed to reinterpret-, in a determined moment of the logical argumentation, the exact dynamic justification of the special behavior of the bodies with angular momentum
In our opinion, there exists certain evidences in nature which allow us to propose hypothesis which have not been raised in Classical Mechanics. There are some clues to believe that the rotational dynamic classical model for rigid solid bodies has not evolved, when the physics of the twentieth century substantially changed.
We aimed to define with precision these new hypothesis and to develop some new experimental testing which allow to corroborate them, with the goal to enunciate the idealized behavior laws, and based on them, establishing a mathematical model which let us develop a computer aided simulation. Finally and based on the theoretical abstractions, we understand that it is possible to conceive practical applications and develop a new specific technology.
4 Ideas for future workHowever, the existence of these physical and mathematical relations between both simultaneous movements of rotation and orbiting could be proposed intuitively.
Moreover, we can ask if the actual dynamic laws provided to define the behavior of the bodies in the space, have enough precision or they do not reflect exactly all possibilities in our world.
We do not know about other type of analysis which study both simultaneous phenomena and could determine a possible interaction between both phenomena. But the fact is that in universe, all orbiting body has intrinsic rotation.
For this reason, I decided to investigate if any physical correlation between both movements exists and, in that case, I would try to obtain its mathematical formulation.
If it were true, the dynamic laws which have been accepted in order to determine the rotational bodies’ behavior until now, would be insufficient to describe the physical reality of the rotational bodies’ movements exactly.
In our study, we research non inertial systems in order to better understand the behavior of rigid bodies supporting non coaxial and simultaneous rotations. As the result of this analysis, I have suggested new hypotheses which oblige to apply these studies to the theory of fields with the objective to explain the dynamic of those bodies.
With this explanation provided, the observation of the universe caused new questions: its secular dynamic balance does not seem to adapt to a Newtonian physics in which the force cause translational accelerated movements continually. The universe balance and its dynamic, does not seem to sympathize with this conceptual structure of classical mechanics.
The analysis of the superposition and composition of the simultaneous movements were done by Galileo [2] in order to explain the trajectory of a cannonball. The understanding or correct interpretation of the fields superposition with dynamic magnitudes generated by momentums or torques, is essential to analyze these phenomena correctly.
In my studies, I have researched the movement of the objects which are supporting multiple rotations, like the spinning top, the boomerang, the hoop and others whose peculiar behavior has been intriguing to the human mind. I think that the conclusions that I show in my texts, change the rational dynamic bases, and incorporate new significant and high impact criteria in rotational dynamics.
Around 1956, the scientist and professor Miguel Catalán [3] proposed to his students, some conjectures about the rotational dynamic by observing the gyroscope behavior and its reactions [4]. In his text books he proposed a possible correlation between the atom structure and the rotational classical dynamic. These conjectures generated in me a scientific curiosity during many years.[5]
Catalán suggested us to experiment with a gyroscope whose rotor had constant rotation around its Z axis. He invited us to take this device with only one hand and perform the following movements:
The results of these experimental proofs are the following:
1. Translation in any direction:
we take a gyroscope with our hand when it is rotating and we move it in a straight line in different directions, maintaining its axis parallel to Z axis or the principal gyroscope axis. No interaction reaction is produced (Look at Figure 0.1A).
2. Additional rotation around the rotation axis: if the device is also rotated around its rotation Z axis, no interaction appears. (Look at Figure 0.1A)
3. Rotation around other axis: if the gyroscope is rotated around any other axis, a resistance to movement is felt in the hand that supports it. (Look at Figure 0.1B)
After these observations, professor Catalán explained us that, in that moment physics had not the answer for these strange phenomena in which we recognized a force which acts on our hand, generating and evident resistance to displacement when we executed a simple rotational movement around any different axis to Z axis.
Our initial speculation and conjectures appeared for the first time in those years, when we endeavored to understand the true dynamic behavior in the rigid solid bodies in non inertial systems (See figure 0.2):
- Understanding the dynamic universe balance.
- Justifying the simultaneous coexistence in the universe, of orbiting and intrinsic rotation phenomena.
With all these questions in mind, I started in the early eighties of the last century, an exhausting historic study on rotational classical dynamics, to get to know the background and what had been written about the subject and other related issues like inertia, magnitude conservation, etc.
I was able to verify that some studies initiated in the nineteenth century, had been later on forgotten. For example, Esprit Jouffret (1831-1904), in the late nineteenth century,7 had already started to analyze the dynamic of the caused reactions in a particle of a rigid body, when it is supported successive non coaxial torque, and M. Hirn8 had concluded that in these situations, non- homogeneous distributions speeds are generated.
As a result of this historical study, I carried out a compendium of all these studies and an abstract, which I published like a summary book about the rotational classical dynamic.9 In this book the different published works which had referred to rotational bodies during the Physics history, were analyze analyzed and commented.
Later on, I defined some differentiated dynamic hypothesis which suggested a rotational alternative dynamic. From these new hypotheses, I established a new specific mathematical formulation in order to determine the movement equations of these bodies by doing experimental positive testing in order to confirm these initial hypotheses.
Therefore, the origin of the Theory of Dynamic Interactions (TDI), arises from the physical observation and the reflection on the validity of mathematical models which accept the vectorial addition of angular magnitudes, with no differentiation on their priority:
- The mathematical model for the development of classical movement equations admits the differential vectorial addition although the physic rotational non-coaxial phenomena are neither commutative nor associative.
- Classical Mechanics admit, since L. Poinsot [10] (1777-1859), the principle of discriminated superposition between movements of translation and rotation; both for the case of movements as well as for the case of separation of force equations and momenta.
- When submitting bodies which are supporting an angular intrinsic momentum to a new momentum, dynamic interactions are produced which allow a variation of the effect of the torque.
- In bodies with kinetic momentum consisting of intrinsic angular momentum and quantity of movement, when non coaxial momentums are applied, the solid will change its trajectory.
Some behavior laws [12] were deduced and suppositions and application phenomena were conceived in addition to the experimental testing which has been done. With a conceptual structure confirmed, I published a new text [13] in which I showed, for the first time, the developed theory and some scientific articles.[14]
3.How much time spent
Although our physics professor, Miguel Catalán managed to pass on to me this curiosity and the dream excitement of the study of physical phenomena, over sixty years ago; it has been for the last thirty-five years that, I have managed to carry out a systematic investigation with a group of collaborating friends. The purpose has been throughout the better understand of the behavior of rigid solid bodies simultaneously submitted to non-coaxial rotations, over the latest 35 years. This research has allowed us to propose new hypothesis about the field’s theory in order to explain the dynamic behavior of the bodies which support non-coaxial accelerations.
Our initials goals in this project were (look at figure 3):
- Analysis of non-inertial systems.
- Research on Fields Theory.
- Study of the behavior of rigid bodies exposed to simultaneous non-coaxial rotations.
- Study of the inertial reactions of the mass exposed to multiple non-coaxial rotations and gyroscopic momentum.
In our experimental line and logical argumentation, in the first place we studied the concept of rotation in Classical Mechanics, but also in Einstein's studies and in the modern theories of relativity. Upon completion of this analysis, we concluded that proposing new specific hypothesis for the movements of the intrinsic rotational bodies is possible. For that purpose, we only needed to reinterpret-, in a determined moment of the logical argumentation, the exact dynamic justification of the special behavior of the bodies with angular momentum
In our opinion, there exists certain evidences in nature which allow us to propose hypothesis which have not been raised in Classical Mechanics. There are some clues to believe that the rotational dynamic classical model for rigid solid bodies has not evolved, when the physics of the twentieth century substantially changed.
We aimed to define with precision these new hypothesis and to develop some new experimental testing which allow to corroborate them, with the goal to enunciate the idealized behavior laws, and based on them, establishing a mathematical model which let us develop a computer aided simulation. Finally and based on the theoretical abstractions, we understand that it is possible to conceive practical applications and develop a new specific technology.
We have proposed a conceptual structure for a new Rotational Interactions Dynamic which is
applicable to bodies exposed to multiples and successive torque.
The initial hypotheses have been confirmed with the studies and experimental testing, and with a
new mathematical model which enable us to simulate the real behavior of bodies exposed to these stimulations. This has allowed us to propose the existence of some general behavior laws in
rotational dynamic, different to those of translational dynamic laws.
A clear correlation has been obtained, among the initial conjectures, the hypothesis, the simulation mathematical
model, the behavior deducted laws, the experimental texting done and the mathematical model
corresponding to the equations of movement which are the result of the proposed dynamic laws.
As we have indicated, our proposals aim at being coherent with the dynamic defined by the theory of
relativity, but we understand that, our conclusions, could be applied also in a revision of theory
relativity [16] too.
The hypothesis and axioms which constitute our proposal, explain the initial question about the
simultaneous rotation and orbiting movements of the celestial bodies in the universe and provide
explanations about the behavior of the general celestial bodies. They will also let us justify other
dynamic phenomena which are poorly understood in this moment, for example the flight of the
boomerang [17][18]. Some articles were published about the flight of the boomerang with an explanatory
video of the proposal theory.[19]
We have also published some works about the application of the TDI in the Dynamic
Interaction Confinement [20] in the fusion nuclear reactors, in the study of satellite trajectories, like the article called Dynamics anomalies in the Pioneer space probes [21], or, in the analysis of the atmospheric phenomena with rotation [22]. In these cases, we understand that important improvements can be obtained based on the dynamic hypothesis proposed.
The dynamic proposals allow to understand some effects of the rotational bodies easily, like
dynamic interactions. The applications of these dynamic hypothesis to other physics and
technology stages, probably allow new and suggestive research advances.
The possible application of this alternative physic-mathematical model to rotational bodies and
systems in astrophysics and astronautics deserve special mention, because new dynamic
hypothesis of work can be achieved.
Also the development of a specific technology, like a dynamic lever, energy saving systems or new ship steering systems for ships is possible.
There exist therefore ample fields of study in science and technology in this new focus of rotational
dynamic.
5. Publishing experience
In 2013 Global Journal of Researches in Engineering, published an article about the theory called: Technological Applications of the New Theory of Dynamic Interactions.
In 2013 Global Journal of Researches in Engineering, published an article about the theory called: Technological Applications of the New Theory of Dynamic Interactions.
It can be consulted in:
https://globaljournals.org/GJRE_Volume13/E-Journal_GJRE_(G)_Vol_13_Issue_5.pdf.
The experience of this publication was really interesting because the journal demanded different and strict control requirements before the edition.
The experience of this publication was really interesting because the journal demanded different and strict control requirements before the edition.
In this text some technological applications of the theory are proposed, for example, the feared
Rollo Coupling of the planes. It happens when a plane, which is flying a screw or any other kind of
air acrobatics which implies, for example, a turn around its main inertia axe, starts a new steering
manoeuvre with curved trajectory.
6. Related media links
Some videos [24] have been created in order to present this theory. In addition, some confirmation
experiments [25] have been performed, and, some experimental videos on the gyroscopic pendulum [26]
or spinning top [27] have been completed and they are been able to watch. [28] These
experiments and the studies done, have been published in articles in specialized journals conference papers.
Other experiments have been also done for other independent [29] researches. There is access to these other experiments in the Internet. [30]
The resultant deductions of this rotational dynamic can be relevant to solve general dynamic
problems, but also to astrophysics and cosmos dynamic. [31] In the universe, the common and
repeated movements of the celestial bodies are accelerated by rotations, except in the case of freefall.
Nevertheless the Einstein theory general of relativity, the special relativity, the Galileo- Newton
relativity and in general Classical Mechanics, are based on the translational relativity proposal.
These theories considered transformations in translational holonomous coordinates, therefore,
when they have rotational movements, angular transformation coordinates should add to the
translational coordinates transformations.
The Theory of Dynamic Interaction allows to identify in a more real and simple way the behavior of
the rotational and orbiting celestial bodies.
In the standard cosmological model there exists a widespread work field in relativistic mechanics by applying a rotational, Theory of Relativity [32], which would allow to better understand the behavior of the Universe.
In the observation of the dynamic systems with simultaneous intrinsic rotation and orbiting, we can
deduce the possible existence of dynamic interactions.
The Theory of Dynamic Interactions allows us to better understand the universe balance to
conceive the true galaxy dynamic and to know why the plane of the ecliptic or Saturn rings33 exists.
In this way, we deduce that the ring in the planetary systems which are generated in the planetary
systems can be the answer of the effect of a torque in the stage of this theory.
As we have explained, the theory gives us an answer to our initial question: check and understand the
physical and mathematical relation between the orbiting and the intrinsic rotation, and therefore,
the cause of the days and night in the Earth, because in this theory a correlation exists between the
rotation and orbiting in the Earth.
The theory defines a universe model in which the forces do not always create accelerations
proportional to the mass. It would mean the existence of increasing speeds to infinity for a constant
force. Instead of that, the force momentum generate a dynamic balance transforming lineal speeds
into orbital speed, and straight trajectories into curve and orbiting paths, by associating dynamic
effects to the speed. In change, Classical Mechanics according to Newton second law, the
dynamic causes are identified with speeds changes.
All celestial mechanics considerations have allowed me to publish a book in two volumes about the
human knowledge evolution: Imago Universi: A human conception of the cosmos. It is a book
about cosmological history, where the last chapter incorporates applications of the Theory of
Dynamic Interactions (TDI) to universe dynamic phenomena. We have completed an introduction video [34]
about the book and a web.[35]
The last chapter of the book Imago Universi shows our paradigm like a new
conceptual structure in order to explore the non-inertial mechanics and, specifically, the celestial
dynamic of rigid bodies under multiple non-coaxial rotations. Later, and as a summary of “Imago
Universi”, I have published an article [36], where an approximation to the Theory of Dynamic
Interactions application can be analyzed in order to better understanding our cosmological
environment and celestial mechanics.
To get more information about the Theory of Dynamics Interactions, some webs can be consulted:
7. Short Biography
I finished the studies of Industrial Engineering in 1964, the Degree in Physical Science in 1968 and
the Doctorate in Industrial Engineering in 1973. Through an exam for Public Service, I entered in
the Administration as an Industrial Engineering serving the Public sector in 1971. In 1977 went
to Financial and Tax Inspector and to State Finance Inspector in 1984.
Initially I worked as an engineer and consultor engineer in Facilities Engineering.
As a civil servant, I was Inspector Chief Inspector in various revenue offices. Also, I was
Subdirector General of the Data Processing Center of the Ministry of Finance (1980 – 1982).
I was member of the international committee of the American Society for heating, refrigerating and
air conditioning (ASHRAE), founder and president of the Technical Spanish Association of Air
conditioning and Refrigerating (ATECYR). I was President of the Associations Federation of
Industrial Engineers, vice-president of the Engineering of Spain Institute and member of their
Energy Commission (1975 – 1980), founder and president of the Professional Association of
Financial and Tax Administration (APIFE). I was Ph.D Professor in the Universidad Politécnica de
Madrid and other educational institutions.
I have published some books: “AIR CONDITIONING” which was prize for the best book of
Aggrupation of Bilbao (ANII) Ed. Dossat (1969), “MODELS OF MANEGERAL BEHAVIOR” APD
(1973), “THE ENERGY AND ITS SOCIAL IMPACT” Ed. INDEX (1976), “THE ENERGY IN
EDIFICATION” ATECYR (1978), “THE ADMINISTRATION OF TAXES IN THE UNITED STATES”,
Institute of Fiscal Studies, Ministry of Economy and Finance (1982), “DICTIONARY OF
MANAGEMENT PROCEDURE AND TAX INSPECTION”. School of Financial and Tax Inspection.
Ministry of Economy and Finance (1984), co-author of: “PLANNING AND STRATEGY TO THE
ENERGY CHALLENGE”, prize the best energy book, APD 1982, “DICTIONARY OF TAX
ADMINISTRATION PROCEDURE”, editorial Gaceta Fiscal, (1985), “LEGISLATIVE DICTIONARY
I.V.A”, editorial Gaceta Fiscal. (1986), “SOCIAL WELFARE IN THE COMPANY” (1991), Ed.
Espasa Calpe, “MANUAL TAX ENGINEER” College of Civil Engineers (1992-2007), “TAX
MANUAL ENTREPRENEUR AND PROFESSIONAL” Editorial Colex (1995), “SPECIAL SCHEMES
IN CORPORATE TAX” (1996) Editorial Colex “TAX MANUAL. NEW IRPF” (1999). Editorial Colex.
“THE FLIGHT OF THE BOOMERANG” Editorial Marcombo (2005), “A ROTATIONAL WORLD:
Theory of Dynamics Interactions” Editorial Marcombo (2008), “Mr. CATALÁN” Ed. ADANAE
(2009), “MIGUEL A. CATALÁN: LIFE MEMORY” Editorial Arpegio. (2012), “IMAGO UNIVSERSI:
History of human conception of the Universe” Editorial Arpegio (2013).
I have published more than fifty technical and scientific articles in newspapers and journals of
construction, engineering, installations, fiscal, sciences and technology, in English or Spanish
language.
- Barceló, G.: Analysis of Dynamics Field Systems Accelerated by Rotation. Dynamics of non-inertial
systems. DeMSET-2011 Congress, Miami. USA.
http://www.coiim.es/forocientifico/FORO%20CIENTFICO/Documentos/DeMSET_2011_GBarcelo.pdf - Appell, P.: Traité de Mécanique Rationnelle, Gauthier- Villars, París, 1909.
- Miguel A. Catalán (1894-1957). He was one of the great spectroscopists in the twentieth century. In order to remind him, his name was given to a crater lunar impact, which is located through the southwest tip of the Moon.
- Barceló, G.: El vuelo del bumerang, Ed. Marcombo 2006, Barcelona. Page 256 and also: G. Barceló, Un Mundo en rotación, Editorial Marcombo Barcelona. 2008. Página 56. http://www.dinamicafundacion.com/
- Catalán, M. A. y León, A: La órbita de los átomos fundamentales, en ASEFQ, 21, 162 y 165 de 1923, W. y en: Física y Química (séptimo curso), Madrid, 1945.
- Barceló, G.: Miguel A. Catalán Sañudo. Memoria Viva. Ed. Arpegio. Sant Cugat. 2012 and Barceló, G.: El Sr. Catalán, profesor del Colegio “Estudio” ADANAE, Madrid 2009.
- Jouffret, E: Théorie élémentaire des phénomènes que présentent le giroscopio, La toupie et le proyectil oblongo, Revue d'Artillerie, Berger-Levrault et Gauthier-Villars, París, 1874. También en Gilbert, P.: Problème de la rotación d'un corps solide autour d'un point. P. 316. Annales de la Société Scientifique de Bruxelles, 1876. Referido en Barceló. G.: Un Mundo en Rotación, pág. 208. Marcombo, Barcelona, 2008.
- Hirn. M.: Théorie analytique élémentaire du giroscopio; en los Annales de l'Observatoire de París, t. IX, citado en Gilbert, P.: Problème de la rotación d'un corps solide autour d'un punto. Anales de la société scientifique de Bruxelles. 1876.
- Barceló, G.: El vuelo del Boomerang. Ed. Marcombo: Barcelona, 2006. http://www.dinamicafundacion.com/
- Poinsot, L.: Connaissance des temps. Théorie nouvelle de la rotation des corps. París 1852.
- Barceló, G.: Technological Applications of the New Theory of Dynamic Interactions. Global Journal of
Researches in Engineering: Mechanical and Mechanics Engineering-G, Volume 13, Issue 5, 2013.
https://globaljournals.org/GJRE_Volume13/E-Journal_GJRE_(G)_Vol_13_Issue_5.pdf - Barceló, G.: Theory of Dynamic Interactions: Laws of Motion. WorldJournal of Mechanics, 3, 328-338.
(2013)
http://dx.doi.org/10.4236/wjm.2013.39036 - Barceló, G.: A Rotating World (Un mundo en rotación). 2008, Editorial Marcombo: Barcelona. http://www.dinamicafundacion.com/
- Barceló, G.: Analysis of Dynamic Fields in Non-inertial Systems. World Journal of Mechanics, Vol. 2, No.
3, 2012, pp. 175-180.
http://dx.doi.org/10.4236/wjm.2012.23021 - Cano, J.: The Pendulum of Dynamic Interactions. Journal of Applied Mathematics and Physics, Vol.3 No.9, September 2015, 1186-1198. Published Online: DOI: 10.4236/jamp.2015.39146. http://www.scirp.org/journal/jamp.
- 16 Barceló, G.: On the Equivalence Principle AC-10-A.2.1.1. 61st International Astronautical Congress,
Prague, CZ. Copyright © 2010 by Advanced Dynamics. Published by the American Institute of
Aeronautics and Astronautics,
Inc.
http://www.coiim.es/forocientifico/FORO%20CIENTFICO/Documentos/ON_THE_EQUIVALENCE_P RINCIPLE.pdf - Barceló, G.: Theory of Dynamic Interactions: The Flight of the Boomerang. Journal of Applied
Mathematics and Physics, 2014, 2, 569-580. Published on line in June 2014 in SciRes.
http://www.scirp.org/journal/jamp
http://dx.doi.org/10.4236/jamp.2014.27063 - Barceló, G.: Theory of Dynamic Interactions: The Flight of the Boomerang II. Journal of Applied
Mathematics and Physics, 2015, 3, pp. 545-555. Published Online May 2015 in SciRes.
http://www.scirp.org/journal/jamp
DOI: 10.4236/jamp.2015.35067 . - Sanchez Boyer J.: The Flight of the Boomerang II, Video.
2015
https://www.dropbox.com/s/stng5b2co1441hk/Boomerang_ENG_mini.mp4?dl=0 https://www.youtube.com/watch?v=mGfrGW5fhOg&feature=youtu.be
https://vimeo.com/129383447 - Barceló, G.: Dynamic Interaction Confinement. World Journal of Nuclear Science and Technology Vol.4
No.4, October 29, 2014DOI: 10.4236/wjnst.2014.44031
http://www.scirp.org/journal/PaperInformation.aspx?paperID=51026
http://dx.doi.org/10.4236/wjnst.2014.44031 - Barceló, G.: Anomalies of the Pioneer space probes, (Pioneer Anomaly). (Anomalías dinámicas en las
sondas Pioneer), RSEF’s 2005 biennial, the summary of which was published in the biennial’s Summary
Book,
http://dinamicafundacion.com/wp-content/uploads/2014/02/ANOMAL%C3%8DAS-DIN%C3%81MICAS-EN-LAS-SONDAS-PIONEER1.pdf
http://www.solociencia.com/fisica/teoria-interacciones-dinamicas-justificacion.htm - Barceló, G.: Dynamic Interactions in the Atmosphere. Atmospheric and Climate Sciences. Vol.4 No.5,
November 20, 2014.DOI: 10.4236/acs.2014.45073.
http://www.scirp.org/Journal/PaperInformation.aspx?PaperID=51584#.VHB4YTSG_To
http://dx.doi.org/10.4236/acs.2014.45073 - Barceló, G.: Technological Applications of the New Theory of Dynamic Interactions. Global Journal of
Researches in Engineering: Mechanical and Mechanics Engineering-G, Volume 13, Issue 5,
2013.
https://globaljournals.org/GJRE_Volume13/E-Journal_GJRE_(G)_Vol_13_Issue_5.pdf - 24 Barceló, G.: Theory of Dynamic Interactions. Videos,
2002.
http://www.youtube.com/watch?v=P9hGgoL5ZGk&list=PL3E50CF6AEBEED47B
http://www.youtube.com/watch?v=XzTrGEtJGXU&list=PL3E50CF6AEBEED47B
http://www.youtube.com/watch?v=dtMqGSU9gV4&list=PL3E50CF6AEBEED47B
http://www.youtube.com/watch?v=qK5mW2j2nzU&list=PL3E50CF6AEBEED47B
http://www.youtube.com/watch?v=vSUkd4slHGQ
http://www.youtube.com/watch?v=P9hGgoL5ZGk&feature=c4-overview-vl&list=PL3E50CF6AEBEED47B
- Cano, J.: The Pendulum of Dynamic Interactions. Journal of Applied Mathematics and Physics, Vol.3 No.9, September 2015, 1186-1198. http://www.scirp.org/journal/jamp
- Pérez, L. A. The pendulum of Dynamic Interactions. Video. 2015. vwww.advanceddynamics.net/the-
pendulum-video.
https://www.dropbox.com/s/rrjb1786ub75a8h/PIDing_m.mp4?dl=0 - Pérez, L. A.: The dance of the spinning top. Video, Valladolid,2015.
www.advanceddynamics.net/spinning-top-video/ - Bauluz, E.: Nuevas hipótesis dinámicas. Este video mostraba los experimentos llevados a cabo
Advanced Dynamics S.A. para probar la teoría de interacciones dinámicas:
www.advanceddynamics.net. El video también está disponible en:
http://vimeo.com/62601974y en:
http://dl.dropbox.com/u/48524938/VTS_Ingles.mov;
http://www.youtube.com/watch?v=vSUkd4slHGQ&feature=c4-overview&list=UUgDHgaGi2I2rmZNoanNbVWQ - Pérez, L. A.: New Evidence on Rotational Dynamics. World Journal of Mechanics, Vol. 3, No. 3, 2013,
pp. 174-177, doi: 10.4236/wjm.2013.33016.
http://www.scirp.org/journal/wjmhttp://dx.doi.org/10.4236/wjm.2013.33016 - Pérez, L. A.: Reflecting New Evidence on Rotational Dynamics, 2013. Video
http://vimeo.com/68763196 - Martín Gutiérrez, Almudena. Flight simulator, trip to Saturn. (Simulador de vuelo, viaje a Saturno). E.T.S.I. Aeronáuticos (U.P. Madrid). Degree project. May, 2015.
- Barceló, G.: On Motion, it’s Relativity and the Equivalence Principle. Journal of Modern Physics. Vol.5
No.17, November 14, 2014. DOI: 10.4236/jmp.2014.517180
http://www.scirp.org/Journal/PaperInformation.aspx?PaperID=51422#.VHB0jzSG
http://dx.doi.org/10.4236/jmp.2014.517180 - Barceló, G.: A new rotational dynamics of interactions for the planet Saturn. (Una nueva Dinámica Rotacional de Interacciones para el planeta Saturno), 2006. http://dinamicafundacion.com/wp-content/uploads/2014/02/UNA-NUEVA-DINAMICA-ROTACIONAL-DE-INTERACCIONES-PARA-EL-PLANETA-SATURNO.pdf
- Sanchez Boyer J.: Imago Universi. Video, Madrid, 2013.
https://vimeo.com/62247544 - Barceló, G.: Imago Universi: A Story of the Human Conception of the Cosmos. Ed. Arpegio: Barcelona, 2013.
http://www.editorialarpegio.com/ http://imagouniversi.com/ - Barceló, G.: Proposal of New Criteria for Celestial Mechanics. International Journal of Astronomy and Astrophysics, 3, 385-391. 2013.
http://dx.doi/org/10.4236/ijaa.2013.34044
Research articles
https://globaljournals.org/GJSFR_Volume16/3-The-Dance-of-the-Spinning.pdf
https://globaljournals.org/GJSFR_Volume16/1-Dynamic-Interaction-A-New.pdf
https://globaljournals.org/GJRE_Volume13/5-Technological-Applications.pdf
Published by Global Journals
© Global Journals Official Blog